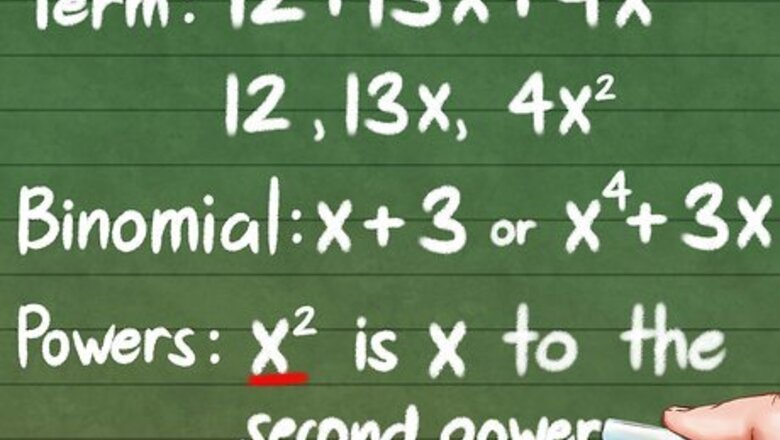
views
Multiplying Two Binomials
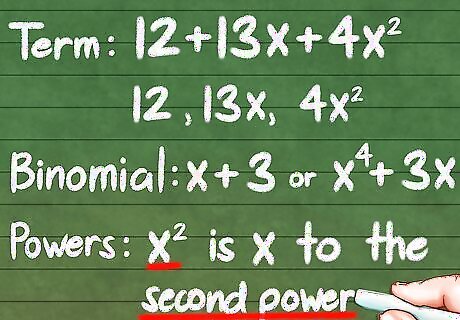
Understand math vocabulary and question types. It will be impossible to solve the questions on your next test if you don't know what they are asking. Luckily, the terminology is not incredibly hard: Terms: A term is simply a part of the equation being added or subtracted. It can be a constant, variable, or both. For example, in 12 + 13x + 4x, the terms are 12, 13x, and 4x. Binomial: This is just a complicated way to say "an expression with two terms," like x+3 or x - 3x. Powers: this refers to an exponent on a term. For example, we could say that x is "x to the second power." Any question asking you to "Find the terms of two binomials (x+3)(x+2)," "find the product of two binomials," or "expand the two binomials" is asking you to multiply binomials.
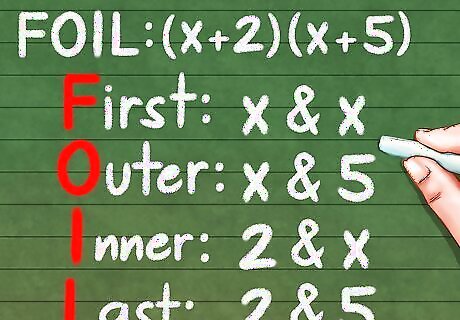
Learn the acronym FOIL to remember the order of binomial multiplication. FOIL is a simple guide for multiplying two binomials. FOIL stands for the order you need to multiply the parts of the binomials together: F is for First, O is for Outer, I is for Inner, and L is for Last. The names refer to the order in which the terms are written. Let's say we are multiplying the binomials (x+2) and (x+5). The terms would be: First: x & x Outer: x & 5 Inner: 2 & x Last: 2 & 5
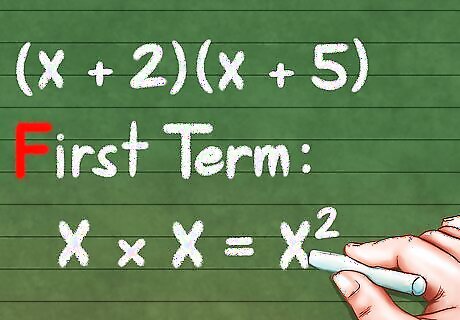
Multiply the FIRST part in each parentheses. This is the "F" of FOIL. In our example, (x+2)(x+5), the first terms are "x" and "x." Multiply these together and jot down the answer: "x." First Term: x * x = x
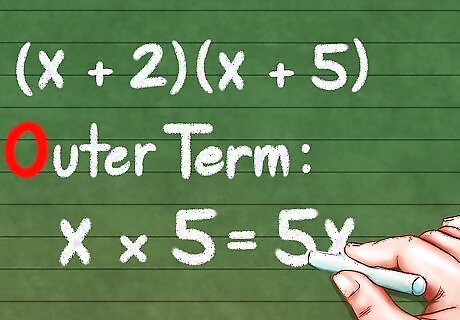
Multiply the OUTER parts in each parentheses. These are two outermost "ends" in our problem. So, in our example (x+2)(x+5), they would be "x" and "5." Together they make "5x" Outer Term: x * 5 = 5x
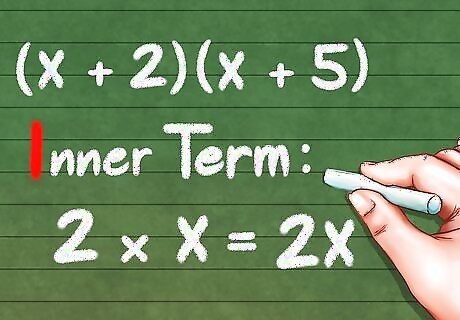
Multiply the INNER parts in each parentheses. The two numbers closest to the center will be your inner term. For (x+2)(x+5), this means you multiply "2" and "x" to get "2x." Inner Term: 2 * x = 2x
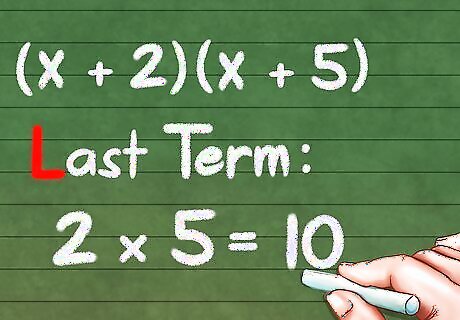
Multiply the LAST parts in each parentheses. This does not mean the last two numbers, but rather the last number in each parentheses. So, for (x+2)(x+5), we multiply the "2" and the "5" to get "10." Last Term: 2 * 5 = 10
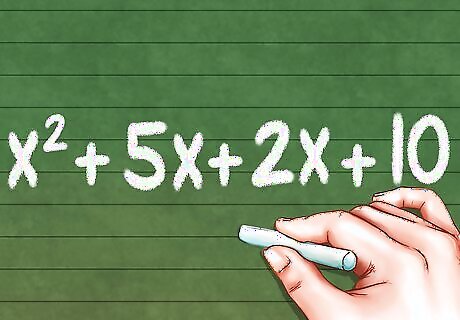
Add all of the new terms together. Combine the terms by adding them together to create a new, larger expression. From our previous example, we get the equation: x + 5x + 2x + 10
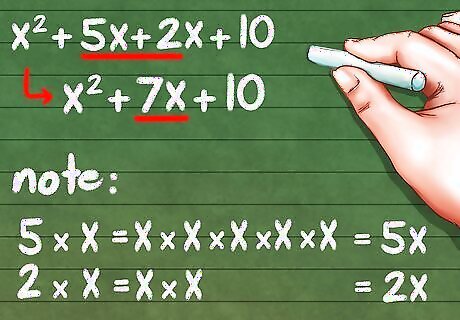
Simplify like terms. Like terms are parts of the equation that have the same variable and power. In our example, the terms 2x and 5x both share an x, and can be added together. No other terms are alike, so they stay put. Final Answer: (x+2)(x+5) = x + 7x + 10 Advanced Note: To learn how like terms work, remember the basics of multiplication. 3 * 5, for example, means that you are adding three fives together to get 15 (5 + 5 + 5). In our equation, we have 5 * x ( x + x + x + x + x) and 2 * x (x + x). If we add all the "x"s in the equation we get seven "x"s, or 7x.
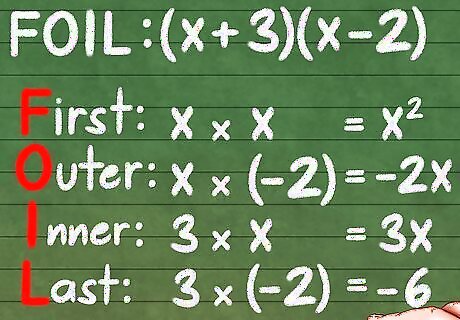
Remember that subtracted numbers are negative. When a number is being subtracted, it is the same as adding a negative number. If you forget to keep the minus sign throughout your calculations you will end up with the wrong answer. Take the example (x+3)(x-2): First: x * x = x Outer: x * -2 = -2x Inner: 3 * x = 3x Last: 3 * -2 = -6 Add all terms together: x - 2x + 3x - 6 Simplify to final answer: x + x - 6
Multiplying More Than Two Binomials
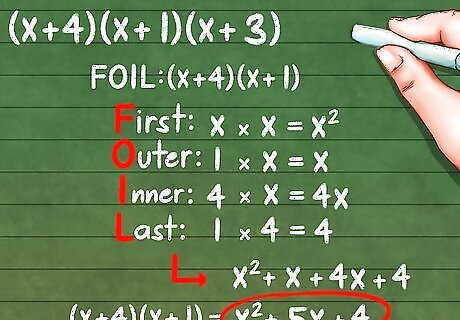
Multiply the first two binomials, temporarily ignoring the third. Take the example (x+4)(x+1)(x+3). We need to multiply the binomials one at a time, so multiply the any two by either FOIL or distribution of terms. Multiplying the first two, (x+4) and (x+1) with FOIL would look like this: First: x*x = x Outer: 1*x = x Inner: 4*x = 4x Last: 1*4 = 4 Combine terms: x + x + 4x + 4 (x+4)(x+1) = x + 5x +4
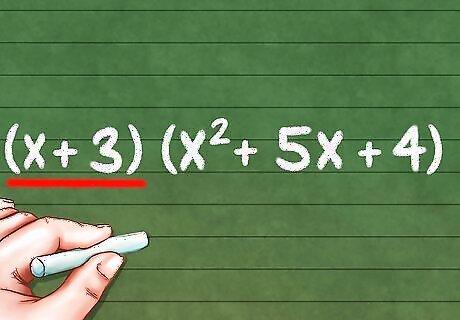
Combine the leftover binomial with your new equation. Now that part of the equation has be multiplied you can handle the leftover binomial. In the example, (x+4)(x+1)(x+3), the leftover term was (x+3). Put it back along with the new equation, giving you: (x+3)(x + 5x + 4).
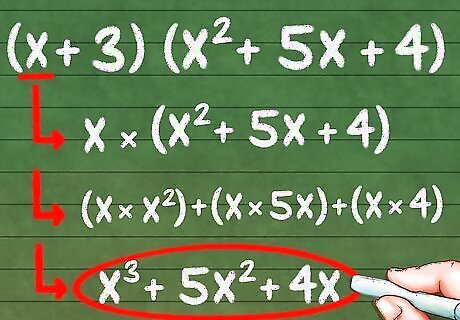
Multiply the first number in the binomial by all three numbers in the other parenthesis. This is distribution of terms. So, for the equation (x+3)(x + 5x + 4), you need to multiply the first x by the three parts of the second parenthesis, "x," "5x," and "4." (x * x) + (x * 5x) + (x * 4) = x + 5x + 4x Write this answer down and save it for later.
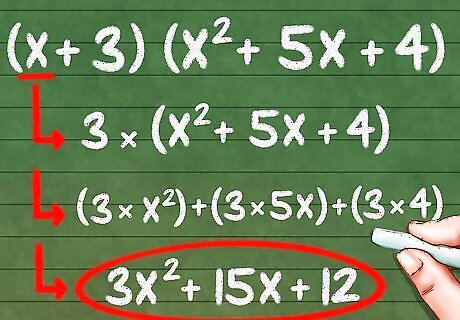
Multiply the second number in the binomial by all three numbers in the other parenthesis. Take the equation, (x+3)(x + 5x + 4). Now, multiply the second part of the binomial by all three parts in the other parentheses, "x," "5x," and "4." (3 * x) + (3 * 5x) + (3 * 4) = 3x + 15x + 12 Write this answer down next to the first answer.
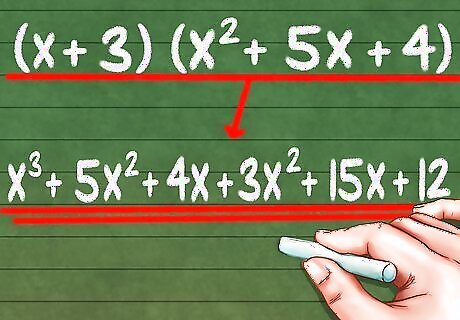
Add together the two answers from multiplication. You need to combine the answers from the previous two steps, as they make up the two parts of your final answer. x + 5x + 4x + 3x + 15x + 12
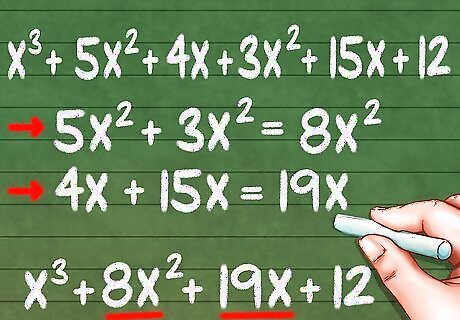
Simplify the equation to get your final answer. Any "like" terms, terms that share the same variable and power (like 5x and 3x), can be added together to make your answer simpler. 5x and 3x become 8x 4x and 15x become 19x (x+4)(x+1)(x+3) = x + 8x + 19x + 12
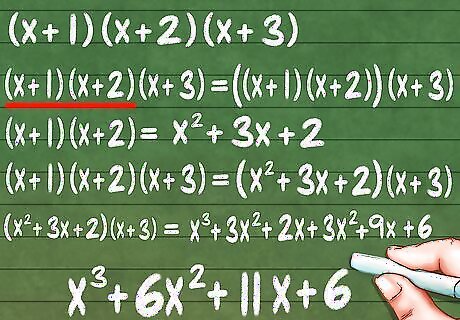
Always use distribution to tackle larger multiplication problems. Since you can use distribution of terms to multiply equations of any length, you now have the tools needed to solve larger problems, like (x+1)(x+2)(x+3). Multiply any two binomials together using either distribution of terms or FOIL, then use the distribution of terms to multiply the final binomial to the first two. In the following example, we FOIL (x+1)(x+2), then distribute the terms with (x+3) to get the final answer: (x+1)(x+2)(x+3) = (x+1)(x+2) * (x+3) (x+1)(x+2) = x + 3x + 2 (x+1)(x+2)(x+3) = (x + 3: + 2) * (x+3) (x + 3x + 2) * (x+3) = x + 3x + 2x + 3x + 9x + 6 Simplify to final answer: x + 6x + 11x + 6
Squaring Binomials
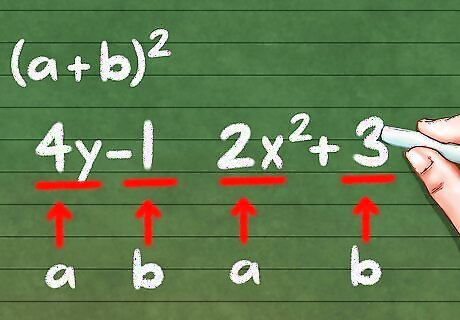
Know how to set up "general formulas." General formulas allow you to simply plug in your numbers instead of calculating FOIL every time. Binomials that are raised to the second power, like (x+2), or the third power, like (4y+12), can be fit into a preexisting formula easily, making solving fast and easy. To find the general formula, we replace all of the numbers with variables. Then, at the end, we can plug our numbers back in to get our answer. Start with the equation (a + b), where: a stands for the variable term (ie. 4y - 1, 2x + 3, etc.) If there is no number, then a = 1, since 1 * x = x. b stands for the constant being added or subtracted (ie. x + 10, t - 12).
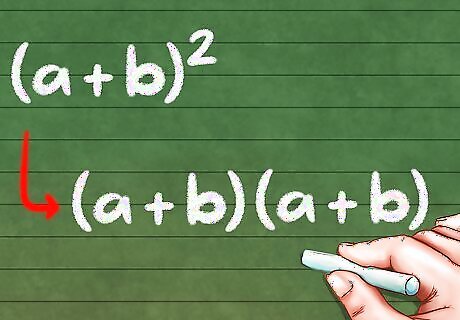
Know that squared binomials can be rewritten. (a + b) may look more complicated than our earlier example, but remember that squaring a number is just multiplying it by itself. Thus, we can rewrite the equation to look more familiar: (a + b) = (a + b)(a + b)
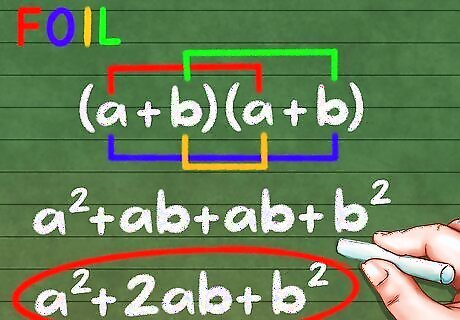
Use FOIL to solve the new equation. If we use foil on this equation we'll get a general formula that looks like the solution to any binomial multiplication. Remember that in multiplication, the order you multiple does not matter. Rewrite as (a+b)(a+b). First: a * a = a Inner: b * a = ba Outer: a * b = ab Last: b * b = b. Add the new terms: a + ba + ab + b Combine like terms: a + 2ab + b Advanced Note: Exponents and radicals are considered to be hyper-3 operations, while multiplication and division are hyper-2. This means that properties of multiplication and division do not work for exponents. (a+b) does not equal to a + b. This is a very common mistake among people.
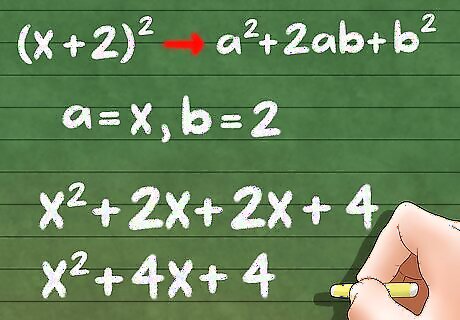
Use the general equation a + 2ab + b to solve your problems. Let's take equation (x+2). Instead of doing FOIL all over again, we can plug in the first term for "a" and the second term for "b", General Equation: a + 2ab + b a = x, b = 2 x + (2 * x * 2) + 2 Final Answer: x + 4x + 4. You can always check your work by performing FOIL on the original equation, (x+2)(x+2). You will get the same answer every time if done correctly. If a term is subtracted, you still have to keep it negative in the general equation.
Remember to insert the entire term into the general equation. Given the binomial (2x+3), you must remember that a = 2x, not simply a = 2. When you have complex terms, you need to remember that both the 2 and the x are squared. General equation: a + 2ab + b Substitute for a and b: (2x) + 2(2x)(3) + 3 Square every term: (2)(x) + 14x + 3 Simplify to final answer: 4x + 14x + 9




















Comments
0 comment